The Official Newsletter of the Washington Science Fiction
Association -- ISSN 0894-5411
Edited by Samuel Lubell samuel@dcez.com
Things That Won't Be In Star Trek: First Contact
A Photo Start to a Pierce-ing Finish
Science Fiction Riddles
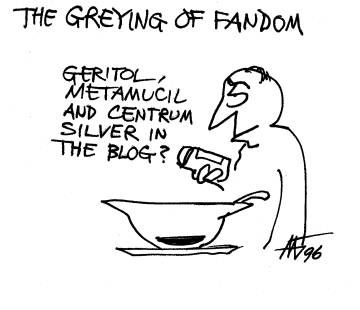
Greying of Fandom
Pál Erdös, An Appreciation by Eric Jablow
Why Not Let The Bad Guys Win One?
3-2-1 Contract: Signed, Sealed, and Delivered
Singing That Old Sweet Song Yet Again
Letter to the Editor
The Last Answer
![]()

Prediction: Things That Won't Be In Star Trek: First Contact
|
|
10. Data getting a job as a stand-up comedian
9. Due to the Disney buy-out of Paramount, every character has three nephews/nieces without having any siblings.
8. The fact that in the last movie the whole crew had been replaced by changelings from Star Trek: Deep Space Nine
7. The bumpersticker on the Enterprise: My other starship is the Death Star
6. In the last movie Picard lost his heir, in the series he had no hair, will this movie mention his pet hare?
5. Data's other brothers: Info, Myth, Rumor, and Lies
4. The missing link between the Borg and Dr. Who's cybermen
3. Troi's driving lessons on the Enterprise
2. Pictures of Voyager on the back of milk cartons
1. Any explanation how any pseudo-military organization could constantly change its whole system of uniforms every other year.
A Photo Start to a Pierce-ing Finish
The 10/4/96 meeting at the Gillilands was called to order at 9:14. The treasurer had $3,355.25 in his coffers. Joe Mayhew announced that he is working on a Disclave History project and is seeking input. He also will schedule a meeting about the future of Disclave. There was a motion made (an actual sign of life). Lee Gilliland proposed that WSFA pay up to $20 to produce Black and White photos of WSFA members who desire such for use by the club. It was passed without objection. There was a suggestion that the photos be taken by a computerized camera so they could be put on the Web.
The meeting was ended at 9:52 followed an election for Disclave 1999 chair. First there was the ceremonial calling of the roll for the election (no bakery products came when called.) Steve Smith acted as honorary trustee (does that imply our other trustees lack honor?) The trustee's slate, Lance Oszko was announced and an alternative, Sam Pierce was nominated and seconded. (No debates were held so neither candidate promised to hold Disclave 1999 on Moon Base Alpha. Although the election was held in Virginia neither candidate spent anywhere near $1 million of his own (or anyone else's) money. There was no negative campaigning (or positive campaigning for that matter.) Neither candidate decided to insult their rival, the media, or the electorate.) After this non-debate and non-campaign, it was decided to hold a secret ballot even though the constitution doesn't require one when only two candidates run. When all the ballots were counted, Sam Pierce became the 1999 Disclave chair. The election was over at 10:07 (Would that other elections be run in this fashion!)
Attending: Pres John Pomeranz, VP Elspeth Burgess, Sec and 98 Chair Joe Mayhew, Treas. Bob MacIntosh, Trustee and 97 Chair Mike Nelson, Trustee. Jim Edwards-Hewitt, Covert Beach, Bernard Bell, Steven desJardins, Chuck Divine, Alexis and Lee Gilliland, Joe Hall, Dan Hoey, Chris Holte, Eric Jablow, Bill Jensen, Judy Kindell, Samuel Lubell, Lance Oszko, Peggy Rae Pavlat, Sam Pierce, Rebecca Prather, George Shaner, Steven Smith, Lee Strong, James Uba, Michael Walsh, Madeline Yeh, George Nelson, Tracy Henry, David Angus.
klmn
1. What is Paul Atreides' least favorite Microsoft Word function?
2. What do you say when your robot commander gives you an order?
3. What do you call a turtle who never lies?
4. What do you do when lying won't get you out of a jam?
|
"I can't turn thirty yet. I haven't seen the Al Jolson Story!"
"Does this mean I'm no longer trustworthy?"
"Are you sure this means I have to become an adult now?"
"Well, I guess I have to put away my Heinlein juveniles."
"How long is the warranty on this body, anyway?"
" |
5. Why did the math teacher always keep the air conditioner on?
Why Not Let The Bad Guys Win One?
Why not let the bad guys win one?
Why should the heroes have all the fun?
Why not let the villains be cool?
It's time to let the Dark Lord rule.
These fantasy quests are becoming trite
Since from page one we know who'll win the fight.
We say this is a magic world where anything can be true.
Yet all the plots are the same in every books in the queue.
|
|
Why not let the bad guys win the war?
Maybe that will keep the book from being a bore.
At least that will be less predictable
Even if the result is less than beautiful
Make that hobbit lose the ring!
Don't find the orb or any magic thing.
Find a different plot, not one already dead
Put this old chestnut back into its bed.
|
|
Have the adventurers try to find a job
Instead of questing for a thing-a-ma-bob
Show the Dark Lord coping with the sewage system
Or let the forces of inflation be what beats him
Have the hero stay an average Joe
Instead of king or wizard or I don't know
Blacken the white and whiten the black
Then the book will have what others lack.
Take it out of times medieval
Make the villain more than a faceless evil
And for a real surprise, let it end
Without sequel or repeat yet again
Why not let the readers win one?
Why should cardboard have all the fun?
Why not let the plot and characters all be new
It's time to let the readers have their due!
Pál Erdös died on Friday, September 20, in Warsaw, Poland. He was 83 years old. There are very few people in this world who can justly be called unique, but Pál was one. People have a stereotype of mathematics and mathematicians; usually their ideas are false. Erdös was the only mathematician I know for whom these ideas are true. No one has ever combined brilliance and eccentricity like him. Certainly no novel or short story has ever presented a character quite like him.
Erdös wrote and co-wrote over 1500 mathematics papers over a 65-year career, and his work inspired hundreds of others. He was a master of number theory, combinatorics, probability theory, and a host of other fields. He invented the field of probabilistic number theory; he first showed what one could do with simple properties of numbers.
His first great result was his elementary proof of the prime number theorem. The prime number function, pi(x) is defined as the number of prime numbers less than or equal to x. The Greeks knew that infinitely many prime numbers exist; the question is how often they occur. Carl Friedrich Gauss observed 200 years ago that
pi(x) ~= x / ln(x),
and conjectured that
lim x->inf pi(x) / (x / ln x) = 1,
but was unable to prove it.
His greatest student, Riemann, worked on this problem, and presented one of his famous but flawed proofs. Riemann considered the zeta function (renamed in his honor),
zeta(s) = 1 + 1/2s + 1/3s + ... Rs > 1,
and defined on the rest of the complex plane by analytic continuation. He then showed how the zeroes of this function are related to the distribution of prime numbers (using Euler's infinite product), and made his famous Riemann hypothesis that all the zeroes of this function in the critical strip 0 < s < 1 occur on the line Rs = 1/2. He showed that this hypothesis implied the prime number theorem, and showed that the hypothesis was plausible. Certainly, he believed it, and we tend to believe it today; no one has ever proved it, though, and the Riemann hypothesis is one of the few great conjectures in mathematics today.
Hadamard and De La Vallèe Poussin proved the prime number theorem about 40 years later, using complex analytic techniques inspired by Riemann's work, but not using it directly. It seems odd that complex numbers need be used to prove a number-theoretic problem, and Erdös was the first person to find a proof not using complex numbers.
Erdös created probabilistic number theory. The name of this field should make you uneasy; certainly the properties of numbers are not truly random. For example, if p > 2 is prime, p + 1 is not. Nonetheless, Erdös was able to demonstrate that many properties of numbers could be treated as random. This led to the Erdös-Kac theorem on the partition function, and many others. Much of modern work on factorization of large numbers depends on his insights.
For example, one important question in computational mathematics today is: "How many prime factors does a typical number have?" More precisely, define the function v(n) as the number of distinct prime factors of n. For example, v(24) = 2, v(30) = 3, and v(7000) = 3. Mathematicians consider the rate of growth of v important because they try to find ways to factor large numbers. The fewer primes that divide a number, the harder it is to factor, and many encryption schemes depend on the fact1 that it is harder to factor a composite number than it is to prove a prime number prime.
In 1920, Hardy and Ramanujan proved the following result using a quite difficult argument. In 1934, Turan found a much simpler one-page proof:
Theorem 1. Let w(n) -> inf arbitrarily slowly. Then the number of x in [1,...,n] such that
|v(x) - ln ln n| > w(n) ln ln n
is o(n).
This is a good result, properly interpreted, but it is hard to work with. It took Erdös and Kac to create the ultimate development of this, and show that the function v(n) essentially behaves like a normal distribution with mean and variance ln ln n:
Theorem 2. Let lambda be a fixed real number. Then,
lim n -> inf 1/n | # { x | 1 <= x <= n, v(x) >= ln ln n + lambda sqrt(ln ln n) }
For example, the average number of prime divisors of integers near 10100 is 5.44, with standard deviation 2.33; even mathematicians find it surprising that about 3% of all 100-digit numbers have only two prime factors.
He was one of the great leaders in graph theory and its sub field of Ramsey theory. The essence of Ramsey theory is contained in the following problem: given six people, either there are three of them who are mutual friends, or there are three of them who are mutual enemies. (Assume there's no middle ground.)
To prove this, choose any one of the people, say Mr. A, and ask whether he has more friends in the group than he has enemies. If he has more friends, he has at least three. Choose any three of them, say Ms. B, Mr. C, and Ms. D. If any two of these three are friendly, then Mr. A and those two form a set of 3 mutual friends. If not, than B, C, and D are mutual enemies. This statement is false for 5 people, and so the Ramsey number R(2;3,3) is 6. That is, if you associate to each group of 2 people one of two properties. You want to be sure either there are 3 people such that each subgroup of two has property A, or there are 3 people whose subsets of 2 have property B. You then need at least 6 people. Similarly, R(2;3,4) = 8. Erdös showed that all these Ramsey numbers are finite, and he extended this to problems of infinite set theory.
I could name many other areas of mathematics that Erdös worked in; however, it would make this note too lengthy. More important is the way he worked. During and after the Los Alamos project, a legend spread that the Hungarian people were actually Martians. After all, they speak a language unrelated to any other on Earth, and they are all incredibly smart. Many of the Los Alamos personnel were Hungarian. Erdös, even though he didn't work there, certainly added support for this conjecture. He was a child prodigy, entered college at 14, received his doctorate at 18, and did only mathematics for the rest of his life.
He was a Jew, even though he was a vocal atheist. As the Nazis came to power, he defiantly proclaimed his Jewishness, and left Europe for America (still remaining an atheist). He became a poor, struggling professor for a while, then a resident at the Institute for Advanced Study, and finally, a nomadic mathematician.
For the last forty years, he had no home, no job, and no interests but math. He wandered the world, visiting math department after math department, rooming with local mathematicians everywhere he went. Other people handled his finances, his taxes, and his travel plans. He co-wrote papers with at least 400 mathematicians, which led to the creation of the Erdös number. If you wrote a paper with him, your number is 1. If you wrote a paper with someone with Erdös number 1, your number is two, and so on. It is estimated that there are a few thousand people with Erdös number 2, and anyone who has an Erdös number at all has it three or less.
Erdös didn't care about money; he made many conjectures and challenges, and issued monetary prizes for each. I have attended a number of his lectures and heard a number of these challenges. I didn't work in those fields, but I do know good problems when I see them, and these were good problems. He was a witty conversationalist, and the star of the post-seminar dinners.
Mathematics is, despite the stereotypes, an intensely social activity. Few mathematicians are solitary, and those who are often are hurt by their solitude. For example, look at Andrew Wiles' proof of the Fermat conjecture, its breakdown, and its repair. Math departments are the most collegial of their universities. People learn more about mathematics at the departmental teas each afternoon than they do in the library. A math department without a popular common room is a substandard department.
Erdös wandered the world. He had no possessions, no money, and no home except for the math departments and the mathematicians' homes of the world. He was always welcome; without warning, he would show up at a colleague's house and announce "My brain is open." He would stay for a week or two, work with his host and others, give talks, eat, and sleep. As he said, "A mathematician is a machine for turning coffee into theorems." Actually in his case, it was coffee and amphetamines.
Pál Erdös spent 65 joyful years creating and co-creating mathematics; the mathematical world shall miss him. I only wish the whole world could understand what it has lost.
* Copyright 1996, the Washington Science Fiction Association. Typeset by LATEX. [ Rendered into HTML by Keith Lynch, November 2002. ]
1 This has not been proven; everyone believes it, but its status is similar to that of the P != NP conjecture.
|
|
In the mysterious absence of
President John Pomeranz, VP Elspeth Burgess led a coup called the
October 18th meeting at the Ginters to order at 9:20. The treasurer reported a balance of
$3,245.80. Disclave '97, Mike Nelson,
announced that the Hotel Contract was signed at the New Carrollton Ramada. Rooms for 1-4 people will cost $76.00. To
celebrate the signing of the contract, there will be an origami party at the November
1st meeting. Secretary Joe
Mayhew will not be at the November 1st meeting. He will find another sucker volunteer
to take minutes.
There was no old business. It was announced that Friday November 29th
(the day after Thanksgiving) would be a fifth Friday. No one volunteered to be a turkey host. The meeting was
closed at 9:45, just as Samuel Lubell walked in.
In attendance were VP Elspeth Burgess, Sec and 98 Chair Joe Mayhew, Treas. Bob MacIntosh, Trustee and 97 Chair Mike Nelson, Covert Beach, Bernard Bell, Chuck Divine, Alexis and Lee Gilliland, Erica Ginter, Bill Jensen, Richard and Nicki Lynch, Barry and Meridel Newton, Lance Oszko, Lotsa Rain, Dick Roepke, George Shaner, Steven Smith, Michael Taylor, Michael Walsh.
Marion Zimmer Bradley's Exile's Song (DAW $21.95 HB, 1996) takes us once again to her planet of Darkover, the world of the Bloody Sun and the scene of about 20 previous novels. While some of the earlier novels in the series (especially Heritage of Hastur and Sharra's Exile) are excellent, her more recent novels have been disappointing - The Heirs of Hammerfell didn't quite ring true and the less said of Rediscovery (co-written by Mercedes Lackey as if both thought the other would do the final draft) the better. I am pleased to say that Exile's Song is better than her last two, although far from the level of the earlier books. This one suffers from some clunky writing, especially at first, although it improves once the initial stage is set.
The key to Darkover's appeal is its mix of fantasy and science-fiction. In the early books this functioned at the level of Boroughs or Brackett in which the characters arrived on the planet by spaceship and then fought the catmen with swords. But this soon deepened so that the major theme is the clash of cultures between the Terrans and their machines and the Darkoverians who have developed mental powers, called laran. Wisely, this conflict did not erupt in war but in political maneuverings and periodic attempts by the Terrans to
understand Darkover. Her favorite plot is that of a Terran (or occasionally a Darkoverian who thinks s/he is Terran) who comes to Darkover, develops his/her laran powers and eventually makes a place for him/herself. She does this in The Shattered Chain and Thendara House, The Bloody Sun, The Spell Sword and The Forbidden Tower,and she manages to do it again here with Margaret Alton, the daughter of Lew Alton, one of the main characters from Heritage of Hastaur and Sharra's Exile. But, since Lew himself is knowledgeable about Darkover and its secrets and the book takes place at the end of the series after generations of Terrans have been on Darkover, maintaining Margaret's innocence requires a heavy authorial hand. This accounts for much of the clunky nature of the early part of the book. (Although her ignorance does provide the author with an excuse to provide enough information to fill in a reader who hasn't read the earlier books.)
Once Margaret has acquired an understanding of the situation and her laran, and is free to be herself, the book becomes better, especially when it focuses on the politics. When Margaret's father left the planet at the end of Sharra's Exile he forfeited his claim to his extensive estate, but not Margaret's claim. Her cousins who had run the estate in her absence are very nervous about her reappearance and are not soothed by her statement that she is now a scholar collecting folk songs and has no knowledge of nor interest in being a heiress on a primitive planet. This family dispute and their efforts to get Margaret to marry one of their two older sons, and her growing friendship with one of the Free Amazons, occupies the better half of the book.
|
|
Surprising, considering the author's age, this book reads more like the beginning of a new trilogy set on Darkover than the conclusion of the saga. The book has a fairly low-key plot, the closest thing to an evil character is defeated half-way through the book, and several incidents/visions promise more to come. Basically, this book seems a way to get Margaret on Darkover for further adventures. I hope we get a chance to see them.
When I read her dedication, my ghostwriter instincts perked up. "For Adrienne Martine-Barnes, who created the character Margaret Alton, and worked on this book with me." Considering Bradley's health and the fact that her last Darkover book was a collaboration (and Mercedes Lackey was widely deemed the heir to the Darkover series before their collaboration came out) one can only wonder how extensive Martine-Barnes' role was in the writing of this novel.
|
|
My recommendation. If you are a Darkover fan (of which there are enough to have their own annual convention) buy it and enjoy. If you are a casual Darkover reader, I'd say read it at the library. If you haven't read Darkover before, this is not the place to start (although the author did provide enough information for the new reader to understand much of what is going on.) I recommend starting with The Shattered Chain or the rewritten version of The Bloody Sun, then moving on to Thendara House, Stormqueen, Heritage of Hastaur and Sharra's Exile.
Solutions to Riddles
1. The UnDune function
2. Aye-Aye robot
3. T'Ruth
4. Then you tell the truth. You're allowed to do that in emergencies. We can't plan for everything you know.
5. So the students could solve cold equations
Hello, this is Terilee. I had a minor correction that I hope you will put in the next WSFA journal.
Jim E-H is listed as not attending the 3rd Friday meeting on the 20th of September. But in fact, he was there and he is mentioned in the minutes of the meeting.
Thanks a lot for taking care of this. Okay, see you at the next meeting.
Ciao, Terilee
Threatened with being assimilated by Windows, the little green men were about to tell our intrepid WSFAns everything. But these revelations are interrupted by the discovery of an alien ship with an English name, the Fibbing Rascal.
"We've got to get back to Earth, we've got a con to run!" said the Disclave '97 chair.
"We can't right now, we've several chapters to go!" said the author.
"Well like man, do what I do. Just flip to last chapter," said Elvis. FLIP!
"I've made contact with the other ship." said the Star Trek fan. "And you won't believe who's on board." The big grin on his face foretold disaster as the main viewscreen sparked into life.
"Klingons?!?" yelled everyone staring at the familiar looking aliens.
"Actually chap," said one of the Klingons. "We are British fans. Can you offer us a lift back to the U.K.?"
"It was all a part of my fiendish plot," said K.O. Pennypitcher, the editor of the Weekly What News. "An alien made contact with me to get ideas on how to sell more newspapers. So we arranged a swap. I took a bunch of aliens from this planet back to Earth to say and do things that make news on which I would have an exclusive. The alien took humans and did the same thing here."
Elvis borrowed the filker's guitar, "You ain't nothing like a news-hound," he began singing.
"But this planet already knew about humans so the bloody bug-eyed monster captured us at a Star Trek convention and made us pretend to be Klingons," revealed the English Klingon.
"It was sort of fun," commented a female in heavy Klingon makeup. "I made lots of progress on the language."
"But we're ready to go home now," the first one said. "Could you beam us aboard or something?"
"Good question," said the WSFA president. He turned to Little Green Men. "Get them aboard or we will let Bill Gates assimilate your planet!"
"By the Thingamobota of Utaljov," one alien swore. "This is real life not Star Trek. We're limited by the laws of physics. Getting them up here will take days."
"Ahem," said Elvis, waving a stack of WSFA Journals. "Let's just skip another chapter or two, shall we?" FLIP!
And so the WSFANs rescued the British and forced the aliens to take them home. Immediately on returning to Earth, the first thing they all did was phone book agents and try to market their story.
"Yeah sure," all the agents said. "You're science fiction fans and you expect us to believe this?" That ended the WSFAns' dreams of money and glory. At least, it did until their next adventure when... but that would be telling.